Suspension is the system of tires, tire air, springs, shock absorbers and linkages that connects a vehicle
to its wheels and allows relative motion between the two. Suspension systems must support both road
holding/handling and ride quality, which are at odds with each other. The tuning of suspensions involves
finding the right compromise. It is important for the suspension to keep the road wheel in contact with the
road surface as much as possible, because all the road or ground forces acting on the vehicle do so through
the contact patches of the tires. The suspension also protects the vehicle itself and any cargo or luggage
from damage and wear. The design of front and rear suspension of a car may be different.
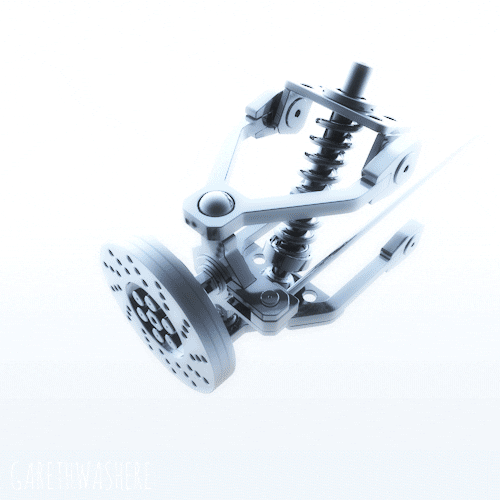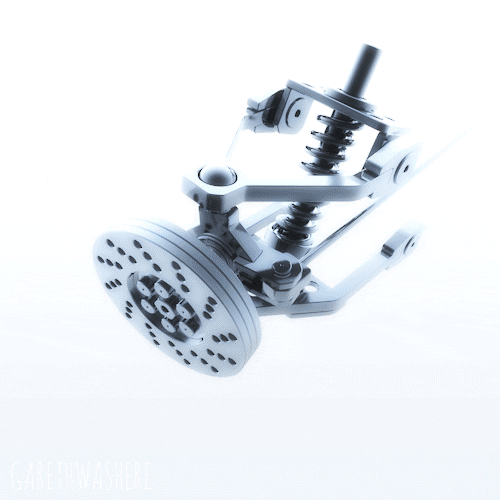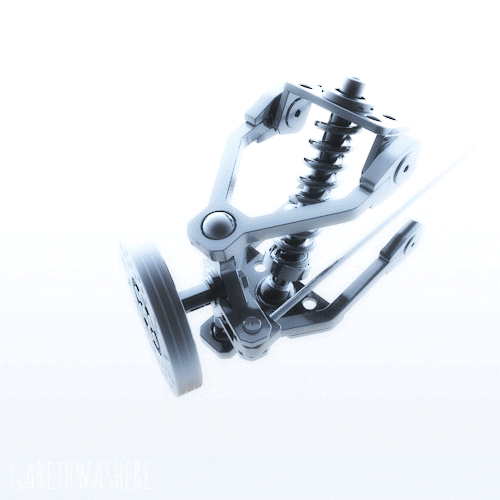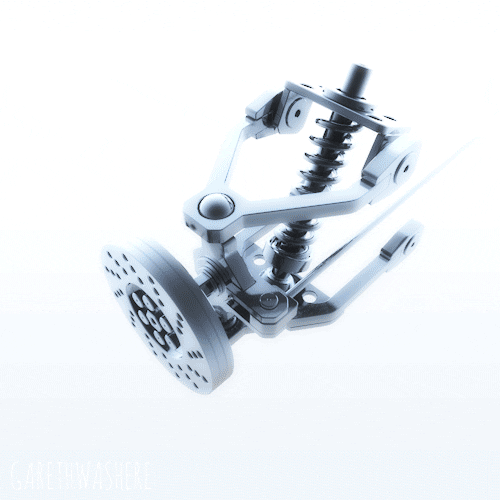
Wishbone Suspension working depicted.
Spring, wheel, and roll rates :
Spring rate :
The spring rate (or suspension rate) is a component in setting the vehicle's ride height or its location in the suspension stroke. When a spring is compressed or stretched, the force it exerts, is proportional to its change in length. The spring rate or spring constant of a spring is the change in the force it exerts, divided by the change in deflection of the spring. Vehicles that carry heavy loads, will often have heavier springs to compensate for the additional weight that would otherwise collapse a vehicle to the bottom of its travel (stroke). Heavier springs are also used in performance applications, where the loading conditions experienced are more significant.
Springs that are too hard or too soft cause the suspension to become ineffective – mostly because they fail to properly isolate the vehicle from the road. Vehicles that commonly experience suspension loads heavier than normal, have heavy or hard springs, with a spring rate close to the upper limit for that vehicle's weight. This allows the vehicle to perform properly under a heavy load, when control is limited by the inertia of the load. Riding in an empty truck meant for carrying loads can be uncomfortable for passengers, because of its high spring rate relative to the weight of the vehicle. A race car could also be described as having heavy springs, and would also be uncomfortably bumpy. However, even though we say they both have heavy springs, the actual spring rates for a 2,000 lb (910 kg) racecar and a 10,000 lb (4,500 kg) truck are very different. A luxury car, taxi, or passenger bus would be described as having soft springs, for the comfort of their passengers or driver. Vehicles with worn-out or damaged springs ride lower to the ground, which reduces the overall amount of compression available to the suspension, and increases the amount of body lean. Performance vehicles can sometimes have spring rate requirements other than vehicle weight and load.
Wheel rate :
Wheel rate is the effective spring rate when measured at the wheel, as opposed to simply measuring the spring rate alone.
Wheel rate is usually equal to or considerably less than the spring rate. Commonly, springs are mounted on control arms, swing arms or some other pivoting suspension member. Consider the example above, where the spring rate was calculated to be 500 lbs/inch (87.5 N/mm), if one were to move the wheel 1 in (2.5 cm) (without moving the car), the spring more than likely compresses a smaller amount. If the spring moved 0.75 in (19 mm), the lever arm ratio would be 0.75:1. The wheel rate is calculated by taking the square of the ratio (0.5625) times the spring rate, thus obtaining 281.25 lbs/inch (49.25 N/mm). Squaring the ratio is, because the ratio has two effects on the wheel rate: the ratio applies to both the force and the distance traveled.
Wheel rate on independent suspension is fairly straightforward. However, special consideration must be taken with some non-independent suspension designs. Take the case of the straight axle. When viewed from the front or rear, the wheel rate can be measured by the means above. Yet, because the wheels are not independent, when viewed from the side under acceleration or braking, the pivot point is at infinity (because both wheels have moved) and the spring is directly inline with the wheel contact patch. The result is often, that the effective wheel rate under cornering is different from what it is under acceleration and braking. This variation in wheel rate may be minimised by locating the spring as close to the wheel as possible.
Wheel rates are usually summed and compared with the sprung mass of a vehicle to create a "ride rate" and the corresponding suspension natural frequency in ride (also referred to as "heave"). This can be useful in creating a metric for suspension stiffness and travel requirements for a vehicle.
Roll rate :
Roll rate is analogous to a vehicle's ride rate, but for actions that include lateral accelerations, causing a vehicle's sprung mass to roll. It is expressed as torque per degree of roll of the vehicle sprung mass. It is influenced by factors including but not limited to vehicle sprung mass, track width, CG height, spring and damper rates, roll centre heights of front and rear, anti-roll bar stiffness and tire pressure/construction. The roll rate of a vehicle can, and usually, does differ front-to-rear, which allows for the tuning ability of a vehicle for transient and steady-state handling. The roll rate of a vehicle does not change the total amount of weight transfer on the vehicle, but shifts the speed and percentage of weight transferred on a particular axle to another axle through the vehicle chassis. Generally, the higher the roll rate on an axle of a vehicle, the faster and higher percentage the weight transfer on that axle.
Roll couple percentage :
Roll couple percentage is a simplified method of describing lateral load transfer distribution front to rear, and subsequently handling balance. It is the effective wheel rate, in roll, of each axle of the vehicle as a ratio of the vehicle's total roll rate. It is commonly adjusted through the use of anti-roll bars, but can also be changed through the use of different springs.
Weight transfer :
Weight transfer during cornering, acceleration, or braking is usually calculated per individual wheel, and compared with the static weights for the same wheels.
The total amount of weight transfer is only affected by four factors: the distance between wheel centers (wheelbase in the case of braking, or track width in the case of cornering), the height of the center of gravity, the mass of the vehicle, and the amount of acceleration experienced.
The speed at which weight transfer occurs, as well as through which components it transfers, is complex, and is determined by many factors; including, but not limited to: roll center height, spring and damper rates, anti-roll bar stiffness, and the kinematic design of suspension links.
In most conventional applications, when weight is transferred through intentionally compliant elements, such as springs, dampers, and anti-roll bars, the weight transfer is said to be "elastic", while the weight which is transferred through more rigid suspension links, such as A-arms and toe links, is said to be "geometric".
Unsprung weight transfer :
Unsprung weight transfer is calculated based on weight of the vehicle's components that are not supported by the springs. This includes tires, wheels, brakes, spindles, half the control arm's weight, and other components. These components are then (for calculation purposes) assumed to be connected to a vehicle with zero sprung weight. They are then put through the same dynamic loads.
The weight transfer for cornering in the front would be equal to the total unsprung front weight times the G-force times the front unsprung center of gravity height divided by the front track width. The same is true for the rear.
Sprung weight transfer :
Sprung weight transfer is the weight transferred by only the weight of the vehicle resting on its springs, and not by total vehicle weight. Calculating this requires knowing the vehicle's sprung weight (total weight less the unsprung weight), the front and rear roll center heights, and the sprung center of gravity height (used to calculate the roll moment arm length). Calculating the front and rear sprung weight transfer will also require knowing the roll couple percentage.
The roll axis is the line through the front and rear roll centers that the vehicle rolls around during cornering. The distance from this axis to the sprung center of gravity height is the roll moment arm length. The total sprung weight transfer is equal to the G-force times the sprung weight times the roll moment arm length divided by the effective track width. The front sprung weight transfer is calculated by multiplying the roll couple percentage times the total sprung weight transfer. The rear is the total minus the front transfer.
Jacking forces :
Jacking forces are the sum of the vertical force components experienced by suspension links. The resultant force acts to lift the sprung mass, if the roll center is above ground, or compress it, if underground. Generally, the higher the roll center, the more jacking force is experienced.
Spring rate :
The spring rate (or suspension rate) is a component in setting the vehicle's ride height or its location in the suspension stroke. When a spring is compressed or stretched, the force it exerts, is proportional to its change in length. The spring rate or spring constant of a spring is the change in the force it exerts, divided by the change in deflection of the spring. Vehicles that carry heavy loads, will often have heavier springs to compensate for the additional weight that would otherwise collapse a vehicle to the bottom of its travel (stroke). Heavier springs are also used in performance applications, where the loading conditions experienced are more significant.
Springs that are too hard or too soft cause the suspension to become ineffective – mostly because they fail to properly isolate the vehicle from the road. Vehicles that commonly experience suspension loads heavier than normal, have heavy or hard springs, with a spring rate close to the upper limit for that vehicle's weight. This allows the vehicle to perform properly under a heavy load, when control is limited by the inertia of the load. Riding in an empty truck meant for carrying loads can be uncomfortable for passengers, because of its high spring rate relative to the weight of the vehicle. A race car could also be described as having heavy springs, and would also be uncomfortably bumpy. However, even though we say they both have heavy springs, the actual spring rates for a 2,000 lb (910 kg) racecar and a 10,000 lb (4,500 kg) truck are very different. A luxury car, taxi, or passenger bus would be described as having soft springs, for the comfort of their passengers or driver. Vehicles with worn-out or damaged springs ride lower to the ground, which reduces the overall amount of compression available to the suspension, and increases the amount of body lean. Performance vehicles can sometimes have spring rate requirements other than vehicle weight and load.
Wheel rate :
Wheel rate is the effective spring rate when measured at the wheel, as opposed to simply measuring the spring rate alone.
Wheel rate is usually equal to or considerably less than the spring rate. Commonly, springs are mounted on control arms, swing arms or some other pivoting suspension member. Consider the example above, where the spring rate was calculated to be 500 lbs/inch (87.5 N/mm), if one were to move the wheel 1 in (2.5 cm) (without moving the car), the spring more than likely compresses a smaller amount. If the spring moved 0.75 in (19 mm), the lever arm ratio would be 0.75:1. The wheel rate is calculated by taking the square of the ratio (0.5625) times the spring rate, thus obtaining 281.25 lbs/inch (49.25 N/mm). Squaring the ratio is, because the ratio has two effects on the wheel rate: the ratio applies to both the force and the distance traveled.
Wheel rate on independent suspension is fairly straightforward. However, special consideration must be taken with some non-independent suspension designs. Take the case of the straight axle. When viewed from the front or rear, the wheel rate can be measured by the means above. Yet, because the wheels are not independent, when viewed from the side under acceleration or braking, the pivot point is at infinity (because both wheels have moved) and the spring is directly inline with the wheel contact patch. The result is often, that the effective wheel rate under cornering is different from what it is under acceleration and braking. This variation in wheel rate may be minimised by locating the spring as close to the wheel as possible.
Wheel rates are usually summed and compared with the sprung mass of a vehicle to create a "ride rate" and the corresponding suspension natural frequency in ride (also referred to as "heave"). This can be useful in creating a metric for suspension stiffness and travel requirements for a vehicle.
Roll rate :
Roll rate is analogous to a vehicle's ride rate, but for actions that include lateral accelerations, causing a vehicle's sprung mass to roll. It is expressed as torque per degree of roll of the vehicle sprung mass. It is influenced by factors including but not limited to vehicle sprung mass, track width, CG height, spring and damper rates, roll centre heights of front and rear, anti-roll bar stiffness and tire pressure/construction. The roll rate of a vehicle can, and usually, does differ front-to-rear, which allows for the tuning ability of a vehicle for transient and steady-state handling. The roll rate of a vehicle does not change the total amount of weight transfer on the vehicle, but shifts the speed and percentage of weight transferred on a particular axle to another axle through the vehicle chassis. Generally, the higher the roll rate on an axle of a vehicle, the faster and higher percentage the weight transfer on that axle.
Roll couple percentage :
Roll couple percentage is a simplified method of describing lateral load transfer distribution front to rear, and subsequently handling balance. It is the effective wheel rate, in roll, of each axle of the vehicle as a ratio of the vehicle's total roll rate. It is commonly adjusted through the use of anti-roll bars, but can also be changed through the use of different springs.
Weight transfer :
Weight transfer during cornering, acceleration, or braking is usually calculated per individual wheel, and compared with the static weights for the same wheels.
The total amount of weight transfer is only affected by four factors: the distance between wheel centers (wheelbase in the case of braking, or track width in the case of cornering), the height of the center of gravity, the mass of the vehicle, and the amount of acceleration experienced.
The speed at which weight transfer occurs, as well as through which components it transfers, is complex, and is determined by many factors; including, but not limited to: roll center height, spring and damper rates, anti-roll bar stiffness, and the kinematic design of suspension links.
In most conventional applications, when weight is transferred through intentionally compliant elements, such as springs, dampers, and anti-roll bars, the weight transfer is said to be "elastic", while the weight which is transferred through more rigid suspension links, such as A-arms and toe links, is said to be "geometric".
Unsprung weight transfer :
Unsprung weight transfer is calculated based on weight of the vehicle's components that are not supported by the springs. This includes tires, wheels, brakes, spindles, half the control arm's weight, and other components. These components are then (for calculation purposes) assumed to be connected to a vehicle with zero sprung weight. They are then put through the same dynamic loads.
The weight transfer for cornering in the front would be equal to the total unsprung front weight times the G-force times the front unsprung center of gravity height divided by the front track width. The same is true for the rear.
Sprung weight transfer :
Sprung weight transfer is the weight transferred by only the weight of the vehicle resting on its springs, and not by total vehicle weight. Calculating this requires knowing the vehicle's sprung weight (total weight less the unsprung weight), the front and rear roll center heights, and the sprung center of gravity height (used to calculate the roll moment arm length). Calculating the front and rear sprung weight transfer will also require knowing the roll couple percentage.
The roll axis is the line through the front and rear roll centers that the vehicle rolls around during cornering. The distance from this axis to the sprung center of gravity height is the roll moment arm length. The total sprung weight transfer is equal to the G-force times the sprung weight times the roll moment arm length divided by the effective track width. The front sprung weight transfer is calculated by multiplying the roll couple percentage times the total sprung weight transfer. The rear is the total minus the front transfer.
Jacking forces :
Jacking forces are the sum of the vertical force components experienced by suspension links. The resultant force acts to lift the sprung mass, if the roll center is above ground, or compress it, if underground. Generally, the higher the roll center, the more jacking force is experienced.